
The gradient is now, which points at an angle toward our front-right. A step to the right also brings us up a little bit, with a slope of 0.5. Ok, but what if we had only turned 45 degrees, so we're at a funny angle on the pyramid? Well, now a step forward brings us up a little bit, but not straight up toward the peak. This makes our gradient, which is a vector pointing to our right, again directly at the peak.
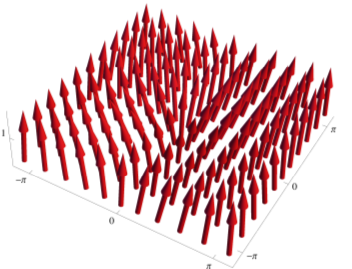
If we step right, the slope must be 1, and if we step forward or backward, there is no slope. This means the peak is now directly to the right. Next, imagine we turn 90 degrees to the left. Then the gradient is the vector, which points straight ahead, toward the peak, which we noticed is the "steepest ascent." This means partial x is 0, and partial y is 1. If we stand so we are facing the peak, then a step forward (+y) has a slope of 1, and a step right or left has no slope.
Now, imagine that from our point of view, taking a step forward is moving in the +y direction, and taking a step to the right is the +x direction. Since the pyramid is smooth, it's easy to realize that it is steepest when you walk directly up toward the peak. We'll also imagine it is perfectly smooth, so it only slopes up toward the peak (for simplicity, we'll say the slope towards the peak is 1). Let's imagine we are climbing up the side of a pyramid in Egypt.


 0 kommentar(er)
0 kommentar(er)
